麻豆淫院

(麻豆淫院)鈥擮ne of the most ambitious endeavors in quantum physics right now is to build a large-scale quantum network that could one day span the entire globe. In a new study, physicists have shown that describing quantum networks in a new way鈥攁s mathematical graphs鈥攃an help increase the distance that quantum information can be transmitted. Compared to classical networks, quantum networks have potential advantages such as better security and being faster under certain circumstances.

"A worldwide quantum network may appear quite similar to the internet鈥攁 huge number of devices connected in a way that allows the exchange of information between any of them," coauthor Michael Epping, a physicist at the University of Waterloo in Canada, told 麻豆淫院. "But the crucial difference is that the laws of quantum theory will be dominant for the description of that information. For example, the state of the fundamental information carrier can be a superposition of the basis states 0 and 1. By now, several advantages in comparison to classical information are known, such as prime number factorization and secret communication. However, the biggest benefit of quantum networks might well be discovered by future research in the rapidly developing field of quantum information theory."

Quantum networks involve sending entangled particles across long distances, which is challenging because particle loss and decoherence tend to scale exponentially with the distance.

In their study published in the New Journal of 麻豆淫院ics, Epping and coauthors Hermann Kampermann and Dagmar Bru脽 at the Heinrich Heine University of D眉sseldorf in Germany have shown that describing physical quantum networks as abstract mathematical graphs offers a way to optimize the architecture of quantum networks and achieve entanglement across the longest possible distances.

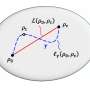
"A network is a physical system," Epping explained. "Examples of a network are the internet and labs at different buildings connected by optical fibers. These networks may be described by mathematical graphs at an abstract level, where the network structure鈥攚hich consists of nodes that exchange quantum information via links鈥攊s represented graphically by vertices connected by edges. An important task for quantum networks is to distribute entangled states amongst the nodes, which are used as a resource for various information protocols afterwards. In our approach, the graph description of the network, which might come to your mind quite naturally, is related to the distributed quantum state."

In the language of graphs, this distributed quantum state becomes a quantum graph state. The main advantage of the graph state description is that it allows researchers to compare different quantum networks that produce the same quantum state, and to see which network is better at distributing entanglement across large distances.

Quantum networks differ mainly in how they use quantum repeaters鈥攄evices that offer a way to distribute entanglement across large distances by subdividing the long-distance transmission channels into shorter channels.

Here, the researchers produced an entangled graph state for a quantum network by initially defining vertices with both nodes and quantum repeaters. Then they described how measurements at the repeater stations modify this graph state. Due to these modifications, the vertices associated with quantum repeaters are removed so that only the network nodes serve as vertices in the final quantum state, while the connecting quantum repeater lines become edges.

In the final graph state, the weights of the edges correspond to the number of quantum repeaters and how far apart they are. Consequently, by changing the weights of the edges, the new approach can optimize a given performance metric, such as security or speed. In other words, the method can determine the best way to use quantum repeaters to achieve long-distance entanglement for large-scale quantum networks.

In the future, the researchers plan to investigate the demands for practical implementation. They also want to extend these results to a newer research field called "quantum network coding" by generalizing the quantum repeater concept to quantum routers, which can make quantum networks more secure against macroscopic errors.

漏 2016 麻豆淫院